Reflections: B. Roy Frieden

Mathematical physicists attempt to understand the laws governing nature by applying mathematics to problems in physics. They create complex formulas that describe the workings of the universe around us — often exploring areas of nature in mathematics that technology so far does not allow us to do in experiments. Typically, they do not conduct experiments or observe nature directly.
In stark contrast to his chosen profession, Professor Emeritus B. Roy Frieden spent many afternoons as a child in Brooklyn, New York, happily exploring the vast acreage of Highland Park — a recreational haven for residents of both Brooklyn and Queens.
Growing up in a popular neighborhood, I had on the order of 100 friends, of all nationalities, all hell-bent on enjoying afternoons playing stickball, handball, and just exploring the park (seeking mischief in its many forms). Virtually penniless — as many were following the years of the Great Depression — I never felt much need for tangible things. If civilization had permitted it, I would have happily stayed in that state of a carefree, sports-minded teenager for the rest of my life.
Roy's father and his father's two brothers owned a mirror-making company, Empire Mirror Works. Unfortunately, it went out of business in about 1948, during a postwar recession. With hopes of joining the family business no longer in his future, Roy felt the need to become more serious at school — becoming a "fierce learner."
Education
In retrospect, Roy feels he benefited greatly from an amazing well-rounded public school system. In addition to traditional core academic subjects — English language arts, math, science and social studies — the curriculum included music appreciation and physical education.
Early on, we were taught music appreciation — listening to and singing the songs of Stephen Foster, spirituals and patriotic songs. We danced minuets and tarantellas. The whole body was involved in the musical experience. In middle school, we graduated to the classics with the works of Rossini, Wagner, Ravel and others. This background in music never left me and led to a lifelong love of classical music. We also had a very effective regime of physical education classes, including mandatory learning to swim (one length of the pool plus a jump into water over one’s head) and boxing with gloves.
Upon graduation from Franklin K. Lane High School, with honors in Spanish and mathematics, Roy enrolled in Brooklyn College in the fall of 1953 — a tradition among his cousins. After receiving a B.S. in Physics, Roy completed phase one of his graduate study, receiving an M.S. in Physics at the University of Pennsylvania in 1959.
At this point, being 22, broke and temporarily burned out academically, I needed a change of lifestyle and, in particular, a job. So, I entered the job market. However, my thesis had been in an esoteric area — expressing the trajectories of elementary particles under a change of coordinate system — and I found that to be of no use when it came to seeking a job. They were all in product development, and I was all thumbs in the lab!
Foray into Industry
Roy's first job was with General Electric, first in Schenectady and then Syracuse, New York. He worked on the initial research phase of a screen-projected color television system for the home. The technology — using a thin deformable pool of oil, through which light was passed — was similar to the Swiss Eidophor projector which provided color transmissions for theaters.
The oil was written line-wise with a scanning electron beam whose instantaneous charge locally deformed the surface of the oil such that it refracted a desired balance of blue, green and red wavelengths. Appropriate optics focused the three beams on a screen. Unfortunately, this large-screen television system was deemed not practical for home use. And, as we are well aware, today’s televisions do not use this principle.
Subsequently, Roy was hired by Bausch & Lomb in Rochester, New York, to work in a computer-oriented thin-film design group, headed by Erwin (Ed) Delano. The aim of the group was to achieve a required energy versus wavelength curve using a given number of planar thin films.
It was believed to be impossible to attain a general curve shape, but I had the honor to witness the solution to the general problem. This was achieved by a colleague of mine, Richard Pegis — using the esoteric mathematics of repeated fractions. In my opinion, Pegis was an absolute genius but, even so, his solution had a drawback due to the required number of films of required indices of refraction. The latter were generally impossible to attain as no natural substance existed with those indices. So, I worked on a 'small change' approach to the design, whereby an initial solution was changed by tiny amounts such that the required energy/wavelength curve was approached by tiny amounts. This is known as the 'Newton-Raphson Method,' and it worked in many cases. I am a longtime fan of Newton-Raphson.
Unfortunately for Roy and the other members of his mathematical optics group, the value of computers for thin-film design had not yet been realized. Machine time was more expensive than the time of the mathematicians and scientists needed to use the machines, and the experience and intuition of skilled designers was considered superior to the computer. Hence, by 1962, the group had been dissolved — and Roy's foray into industry had ended.
Back to Education
Unemployed, Roy made plans to fly back to Brooklyn and, if lucky, land a job as a high school math or physics teacher. But Ed Delano had another idea. Knowing Roy's strengths in mathematical computation and inverse problems, he recommended Roy to a professor at the University of Rochester (UR), Dr. Robert E. Hopkins. Hopkins was searching for someone who could help him write a computer program that would analyze knife-edge intensity data from a given optical system and compute the modulation transfer function.
Dr. Hopkins, aware that I no longer had a firm connection in Rochester, asked me how much longer I intended to stay. I told him, truthfully, 'just till the end of the day!' It was music to my ears when he said, with urgency (paraphrased) 'Don’t go! I need you here. At this point, just buy a Basic Fortran user’s manual and learn how to program.' I gladly did this and, as a test, wrote a Fortran program for computing the sine of an angle. That was the start of my career in image science. Hurray, no more product development! (As a confirmation of my new self-confidence I 'discovered' the Nyquist sampling theorem during development of the knife-edge program. The fact that it was long known to electrical engineers didn't bother me; what mattered was that it was correct and formed the backbone of the program.)
Originally planning to enroll in University of Rochester's Institute of Optics as a master's student, Roy changed his mind and entered the Ph.D. program in the fall of 1963. Three years later, he received his doctorate degree with a dissertation entitled, "Selected Problems in Image Evaluation and Image Synthesis." Two of those problems defined new concepts.
1. I founded the field of 'laser beam shaping.' This accomplishes the design of either a single aspheric surface, or a sequence of axially symmetric refraction surfaces that redistributes the rays of light emerging from a laser, which originally obey a Gaussian distribution in the image plane, into a uniform distribution: see above ref. to 1966 dissertation; or the paper "Lossless Conversion of a Plane Laser Wave to a Plane Wave of Uniform Irradiance," Appl. Opt. 4, 1400-1403 (1965).
2. I conceived of, and analytically derived, a three-dimensional optical transfer function. See ref. "Optical Transfer of the Three Dimensional Object," J. Opt. Soc. Am. 56, 56-66 (1967). This goes as the Fourier transform of the system three-dimensional point spread function. Interestingly, it obeys the autocorrelation of the lens pupil function (which by itself gives the conventional two-dimensional transfer function in the x- and y-component frequencies), but with a further integration along a straight line whose location in the autocorrelation region is linear in the third (z-component) frequency.
The College of Optical Sciences

OSC group photo on September 13, 1967. B. Roy Frieden can be seen in the front row, second from left.
In 1966, fate entered in the form of two independent events to push Roy to make the move to Tucson, Arizona. First, James (Jim) Eyer (then, UR professor and later, first associate director of OSC) talked to him about great new opportunities for people with advanced degrees in optics at the newly-formed University of Arizona’s Optical Sciences Center (OSC). Secondly, fellow UR graduate Robert Fischer (later, the founder and president of Optics 1, Inc.) suddenly appeared with a thick album of personal photographs taken recently in Tucson — showing sites such as the Desert Museum and Kitt Peak National Observatory. Roy was deeply impressed with the beauty of these pictures and with the golden opportunity at OSC for someone with his optics and mathematics background. So, he contacted its founder, Aden Meinel, to apply for a position, and a time was set up for him to interview at OSC in late January 1966.
Arrangements were made for me to stay with Roland and Pam Shack while in Tucson, which turned out to be very fortunate. As a great lover of classical music, I have always needed at least a two-hour 'shot' of it every day — so, I was concerned that Tucson might not have a classical radio station. To my relief, Pam assured me there was one that she and Roland enjoyed very much. Looking back, I now know that radio stations should have been the least of my worries. I should have been worried about the grand trick Mother Nature was playing on all of us easterners visiting out west.
A great snowfall (now known as the 'Blizzard of 1966'), was descending upon the Northeast — dropping nearly 27 inches of snow in a day's time. Airport landing fields, including ones in Rochester and nearby cities of Buffalo and Syracuse were closed. I was able to fly to Chicago's O'Hare airport where I stayed overnight — sleeping in a chair in the waiting area — to wait for airports closer to Rochester to open. The next day I was informed that Rochester's airport was still closed, but the one in Toronto, Canada was open. They anticipated that once I got to Toronto, the New York Central Railroad line — which had a branch around Lake Ontario to Rochester — might be open (although still snowed over at this time). I finally made it to Rochester by this plan.
However, once there, I realized that all the streets and roads were completely snowed over, so I still couldn't make it home. I checked-in to a downtown hotel and tried again the next day. That morning, I found all the major roads (but not the city streets) were cleared to about a six-foot width down the middle and lined with about ten-feet of hard packed snow on each side — forming a vast labyrinth which, once inside, presented only rare opportunities to leave. And, of course, no taxi would (or could) take me home, so I set off on foot to walk the eight miles. I entered the tunnel of snow and, luckily, made it home with no mishap. The next day, Aden called to offer me an assistant professorship at OSC, and I readily accepted!
Academic Career

B. Roy Frieden fielding questions at the UFO Symposium during the OSA Meeting in Tucson, April 5-8, 1971.
For almost 40 years, Roy enjoyed a satisfying career as a teacher and researcher in optical sciences — with applications to image processing, restoration and related subjects. Roy regards his invention of a principle of maximum entropy very important for these purposes — see B. R. Frieden, "Restoring with Maximum Likelihood and Maximum Entropy," J. Opt. Soc. Am. 62, 511-518 (1972).
One picture is worth a thousand words. Maximum entropy reconstruction also was used by astronomers at Kitt Peak National Observatory (KPNO) to enhance images of nebula and galaxies. Dr. Don Wells, a computer-oriented astronomer at KPNO, contacted me for the purpose of applying the maximum entropy approach to such images. In particular, he wanted to enhance the resolution of an atmospherically blurred image of what was called the 'Bird Galaxy.' This is shown in the photo labeled 'Bird.' On the right, is its best entropy-based version (note: vertical shading on far right is due to age of photo). Notice the emergence of a spiral-armed galaxy from the blurred central region of the image. This entropy-based approach had a lot of application to real-world imagery, especially during the Cold War with Russia.
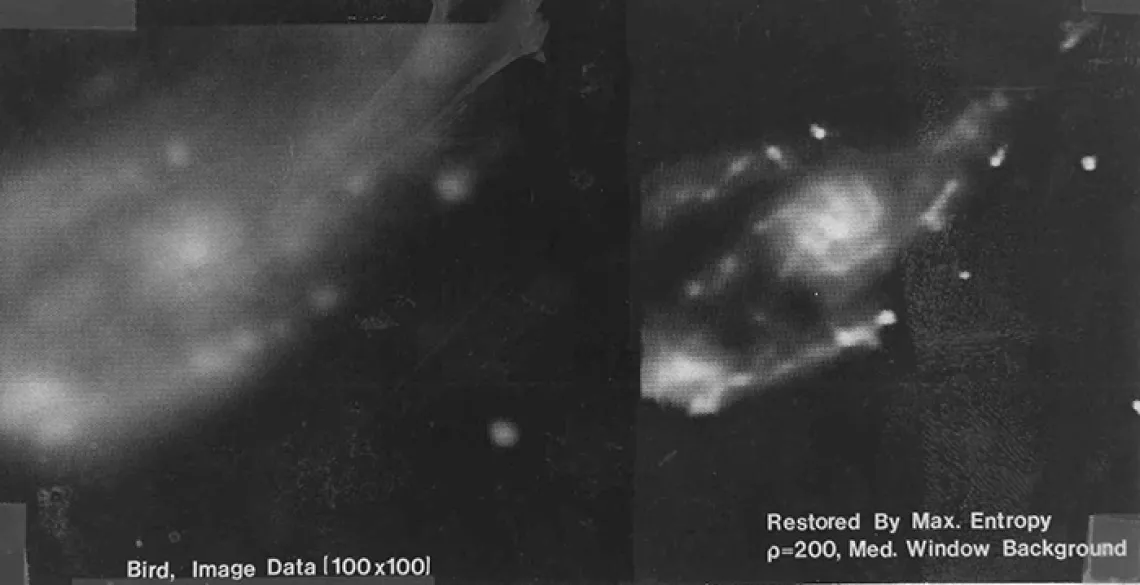
Bird - On the right, is its best entropy-based version (note: vertical shading on far right is due to age of photo). Notice the emergence of a spiral-armed galaxy from the blurred central region of the image.
Retirement
In 2002, Roy retired from OSC as Professor Emeritus. Not the kind of man who enjoys days of simple leisure, he has continued doing mathematically-oriented work — applied to an entirely different field, cell biology. His most recent work has been in collaboration with Dr. Robert (Bob) Gatenby, M.D., Moffitt Cancer Center (formerly, head of the department of radiology at The University of Arizona College of Medicine).
I find it intriguing how the same laws of math and physics that describe optics and image science also describe the motions of proteins through cells and into membranes. For example, Bob and I recently published a finding of how and why enzymes work so well in speeding up reactions. OSC’s former director Dick Powell once said at a faculty meeting, ‘all science is optics.’ At the time I thought this was a stretch, but now see that my background in optical sciences has well-prepared me for this biological work.
Books Authored
- The Computer in Optical Research, Vol. 41 of Topics in Applied Physics, B. Roy Frieden
- Physics from Fisher Information: A Unification, B. Roy Frieden
- Probability, Statistical Optics and Data Testing: A Problem Solving Approach, B. Roy Frieden
- Science from Fisher Information: A Unification, B. Roy Frieden
Books Edited
- Exploratory Data Analysis Using Fisher Information, B. Roy Frieden and Robert A. Gatenby
